Quartic Roots-Employing 2 ‘Big W’ Substitutes | by Greg Oliver | Jun, 2023

Quartics With ‘Big W’ Condition Substitutes For Less complicated Roots vs Consistent E Variations

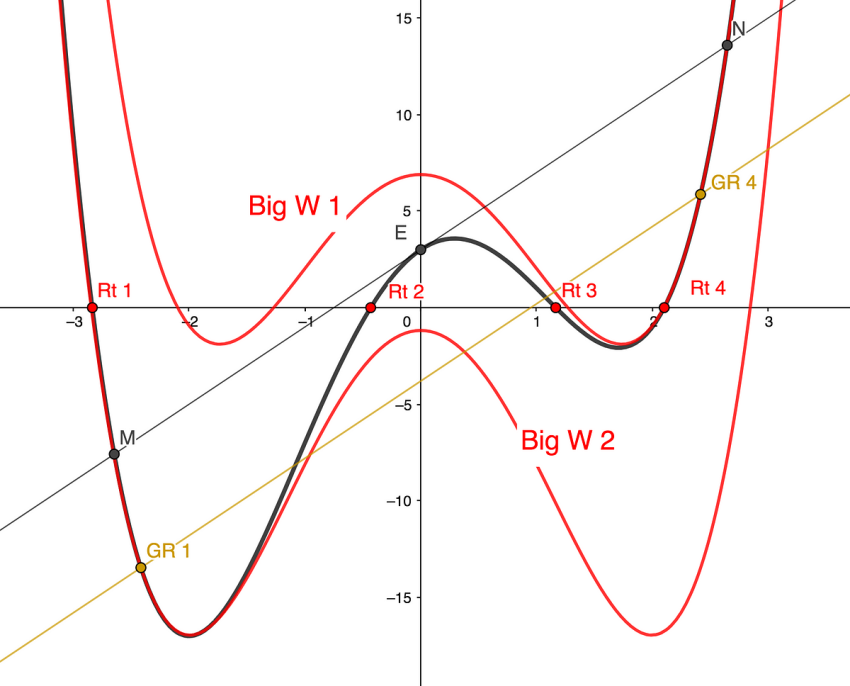
I have beforehand applied Large W’s y=x⁴+Bx²+E as genetic architecture for obtaining Decreased Quartic roots, but this write-up adopts 2 generic Large W’s as substitute profiles for the 2 exterior roots. Feel of it as ‘2 factor’ verification!

While the resultant roots are incredibly shut to precise, the critical edge of the technique, aside from learning Polynomial architecture, is that getting found the conforming Large W’s, it is a extremely uncomplicated matter to recalculate roots with structure improvements in the Regular E.

Derivation of the 2 Huge W substitute functions is made simple with

1. the use of the ‘Golden Ratio’ to present matching intercept information
2. a straight line y=Dx+E equalling linear material of the topic perform, y=x⁴+Cx²+Dx+E yielding, zero regular y=x⁴+Cx²= derived intercepts

Note: in the course of this write-up, except exampled if not, coefficient A of x⁴ is assumed to =+1 as it doesn’t modify roots if other introduced values are divided through the perform.

The put up assumes math at the superior school level.

First, a quick note on Major W and the Golden Ratio.